Difference between revisions of "Busbar"
m (Text replacement - "[[Special:MyLanguage/Series and parallel" to "[[Special:MyLanguage/Series and parallel connections") |
|||
| (6 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
[[Category:Power distribution]] | [[Category:Power distribution]] | ||
| + | <languages /> | ||
| + | <translate> | ||
| + | <!--T:1--> | ||
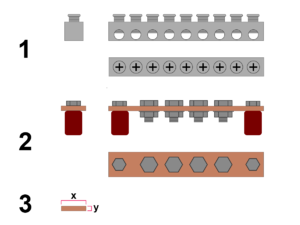
[[File:Busbar2011292.png|thumb|right|''(1),(2)'' - Examples of different busbar designs with different perspectives.<br /> ''(3)'' - The amount of current that a busbar can handle depends upon its thickness.]] | [[File:Busbar2011292.png|thumb|right|''(1),(2)'' - Examples of different busbar designs with different perspectives.<br /> ''(3)'' - The amount of current that a busbar can handle depends upon its thickness.]] | ||
| − | |||
| − | + | <!--T:2--> | |
| + | Busbars are an essential component of electrical systems as they allow safe and easy [[Special:MyLanguage/Series and parallel connections|parallel connections]] of circuits. There are many different designs of busbars that all serve this same purpose. It is important any busbar used is rated to handle the current that it must carry, and that the busbar is properly isolated from all other system components. Busbars should only be installed within enclosures that are protected from unauthorized or accidental access as they have live exposed parts. | ||
| + | <!--T:3--> | ||
| + | The amount of current that a standard copper busbar can carry can be determined by measuring the height and width of the busbar in mm - see ''(3)'' in the graphic. If these two values are multiplied it will give a mm² value that can be used with the table for conductor ampacity from [[Special:MyLanguage/Wire, overcurrent protection, and disconnect sizing and selection|Wire, overcurrent protection, and disconnect sizing and selection]]. | ||
| + | |||
| + | <!--T:4--> | ||
The formula for calculating the cross-sectional area of a busbar is: | The formula for calculating the cross-sectional area of a busbar is: | ||
| − | + | <!--T:5--> | |
| + | {| class="wikitable" border=1 style="width: 60%;" | ||
| + | ! style="width: 30%"|Cross sectional area | ||
| + | ! style="text-align:left;"| = x (width) × y (height) | ||
| + | |} | ||
| + | |||
| + | <!--T:6--> | ||
| + | '''Example 1:''' A busbar is measured to have a height of 5 mm and a width of 10 mm. What is its mm² cross sectional area? At 75°C how much current can it carry? | ||
| + | |||
| + | <!--T:7--> | ||
| + | :Cross sectional area = 5 mm × 10 mm | ||
| + | :Cross-sectional area = 50 mm² | ||
| + | :50 mm² can carry 130 A at 75°C according to the [[Special:MyLanguage/Wire, overcurrent protection, and disconnect sizing and selection#Conductor ampacity table|conductor ampacity table.]] | ||
| − | + | ==Notes/references== <!--T:8--> | |
| − | + | </translate> | |
| − | |||
Latest revision as of 22:15, 1 March 2021
Busbars are an essential component of electrical systems as they allow safe and easy parallel connections of circuits. There are many different designs of busbars that all serve this same purpose. It is important any busbar used is rated to handle the current that it must carry, and that the busbar is properly isolated from all other system components. Busbars should only be installed within enclosures that are protected from unauthorized or accidental access as they have live exposed parts.
The amount of current that a standard copper busbar can carry can be determined by measuring the height and width of the busbar in mm - see (3) in the graphic. If these two values are multiplied it will give a mm² value that can be used with the table for conductor ampacity from Wire, overcurrent protection, and disconnect sizing and selection.
The formula for calculating the cross-sectional area of a busbar is:
| Cross sectional area | = x (width) × y (height) |
|---|
Example 1: A busbar is measured to have a height of 5 mm and a width of 10 mm. What is its mm² cross sectional area? At 75°C how much current can it carry?
- Cross sectional area = 5 mm × 10 mm
- Cross-sectional area = 50 mm²
- 50 mm² can carry 130 A at 75°C according to the conductor ampacity table.